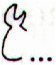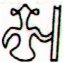Whatever Metoro may have meant at
Cb1-10 with his hiko o tea (not preceded by atua)
we can see a 'fist held high in front' as a sign of 'nothing as
yet counted' = a new beginning:
... The practice of turning down the fingers,
contrary to our practice, deserves notice, as perhaps explaining
why sometimes savages are reported to be unable to count above
four. The European holds up one finger, which he counts, the
native counts those that are down and says 'four'. Two fingers
held up, the native counting those that are down, calls 'three';
and so on until the white man, holding up five fingers, gives
the native none turned down to count. The native is nonplussed,
and the enquirer reports that savages can not count above four
...
|
*AUGUST 14 (*146 = 2 / 5 * 365) |
15
(227) |
16 |
|
'September 24 (84 + 183) |
25 (268) |
26 |
|
October 21 (*214 = *146 + *68) |
22
(295 = 227 + 68) |
23 |
|
Neck-2 (Dragon)
ASELLUS TERTIUS
= κ Bootis,
κ
VIRGINIS,
14 Bootis (214.8) |
Al Ghafr-13 /
Svāti-15 /
TAHUA-TAATA-METUA-TE-TUPU-MAVAE-6
(a
pillar to stand by)
15
Bootis (215.2),
ARCTURUS = α Bootis
(215.4),
ASELLUS SECUNDUS
= ι Bootis (215.5),
SYRMA (Garment's Train) = ι Virginis,
λ Bootis (215.6), η Apodis (215.8) |
ι
Lupi, 18 Bootis (216.3),
KHAMBALIA (Crooked-clawed)
= λ Virginis (216.4), υ Virginis (216.5), ψ
Centauri (216.6), ε Apodis (216.8) |
 |
 |
 |
|
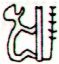
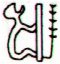
Cb1-5 |
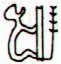
Cb1-6 (398) |
Cb1-7 |
|
rutua |
te pahu |
rutua te maeva |
|
η Arietis (31.9) |
ξ¹ Ceti (32.1) |
θ Arietis (33.3),
MIRA
= ο Ceti (33.7) |
|
*FEBRUARY 12 (408 = 365 + 43) |
13
(*330 = *147 + *183) |
2-14
(45 = 31 + 14) |
|
'March 24 |
Julian equinox |
26 |
|
April 21 (111 = 43 + 68) |
22
(*32 = *215 - *183) |
23 |
|
*AUGUST 20 (232 = 8 * 29) |
|
'September 30 (*193) |
|
October 27 (300 = 232 + 68) |
|
σ
Bootis (220.2), η Centauri (220.4) |
 |
|
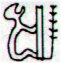
Cb1-11 (403) |
|
ka higa te ao ko te henua ra ma te hoi atua |
|
ν Ceti (37.9) |
|
*FEBRUARY 18 (49 = 7
* 7) |
|
'March 30 (90 = 49 + 41) |
|
April 27 (300 - 183 = 117) |
In order to understand the meaning of 403
(at Cb1-11) this number should be reduced by 4 precessional days
from Gregory XIII up to the time of rongorongo and by
another 4 because he moved the date for spring equinox. 403 - 4 - 4 = 395.
Similarly Cb1-6 (398) should be
understood as representing day 398 - 4 - 4 = 390. And
390 = 13 * 30.
However, we will remember from the
compressed henua calendar in the text on the G tablet that
the total cycle probably was 13 * 31 = 403 days (cfr the
glyph number at Cb1-11):
|







 1 winter solstice
1 winter solstice