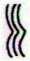|
TRANSLATIONS
|
In the glyph
catalogue I have listed 4 ika hiku glyphs under Q, and
one of them is the 'prototype' I used as an example of the glyph
type:
 |
 |
 |
 |
 |
|
Qa9-36 |
Qb2-15 |
ika hiku |
Qb2-24 |
Qb6-106 |
It is quite
difficult to decide - now several years later - which one it was,
which became the prototype, whether it is Qb2-15 or Qb2-24. They
are drawn exactly alike.
The 'legs' are
definítely not depicting 'feathers' (fire). Those in Qb2-15 and
Qb2-24 are rather meant to allude to the opposite phenomenon, ua
(rain):
 |
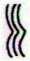 |
|
Qb2-15 |
ua |
It seems as if the
triplet of wave-forms in ua have been turned a quarter into a
horizontal position. Maybe this implies two things: First, that
Qb2-15 is located where the horizontal dimension is ruling (i.e. a
solstice). Secondly, that it is not water in form of rain but water
in form of sea waves.
...
Mermaid's purses
(also known as Devil's Purses) are the egg cases of skates, sharks
and rays. They are among the common objects which are washed up by
the sea. Because they are lightweight, they are often found at the
furthest point of the high tide ...
|
|
The idea of
sea waves in Qb2-15 and Qb2-24 finds support among
the ua glyphs of Tahua:
Though in Aa6-25
and Aa6-35 the waves are, it seems, submerging the entities below.
In Qb2-15 and Qb2-24
the meaning ought to be the opposite of submerging. Having read how
'fire feathers' can be subdued by an object between them and the
observer, it is quite natural to see the same trick used here in order to
illustrate how the waves of the sea are subdued (as when an egg case,
impersonating the sea, is washed high ashore):
 |
|
*Ca14-23 |
In C the winter
solstice sun (at *Ca14-23) is half hidden. But in Qb2-15 another phenomenon
must be depicted, maybe
the change from a season of water to a season of land. If so, then
the time could be say 4 months beyond winter solstice.
We need to
investigate where in the text cycle of Q these two ika hiku
glyphs are located. We need facts.
|
|
The structure
of the Q text has been discussed at ariga erua, and
winter solstice was found to be in line Qb5:
From this it seems to be an easy task to count glyphs and find
where the pair of ika hiku glyphs 'stranded high on the
beach' are located in the calendar cycle.
|
The day of Rogo should
be 363, not 360. I will change the last sentence into:
Q
has
the
arrival of winter solstice 9 days earlier than the
break in the calendar (between *Qb5-35 and
*Qb5-36), but G has it the other
way - with day 400
coming 9 days earlier than Rogo.
From day 363 to day 369 (= 368 + 1) there are 6 days, but 5 days could
be the measure:
| 360 |
361 |
362 |
363 |
364 |
365 |
366 |
367 |
368 |
369 |
|