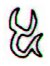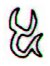Next page:

In Tahua there is a tara glyph which exhibits how the top part can be drawn as a separate unit:   Aa5-14 Aa5-15 Aa5-16 Aa5-17 Aa5-18 The curious back of Aa5-15 resembles that in Eb8-26: Eb8-20 Eb8-21 Eb8-22 Eb8-23 Eb8-24 Eb8-25 Eb8-26 On the other side of Tahua an internal parallel exists, and there we find more evidence (in Ab7-34) of the 'equality' between Aa5-15 and Eb8-26: Ab7-33 Ab7-34 Ab7-35 Ab7-36 Ab7-37 Ab7-38 Ab7-39 These facts should stimulate us to draw conclusions.

Tahua is an extremely long text (1,334 glyphs) and therefore not a good starting point for investigating how an Easter Island calendar can be constructed. The best text for doing so is instead K (probably once 192 glyhs). Earlier (in the pure 'chapter') we have for K established a summer half year with 52 glyphs:   46 51 glyphs Ka2-10 Ka4-14 Ka4-15 *Kb2-15 *Kb2-16 0 47 summer *100 *101 The calendar summer covers 15 periods and the glyphs of summer are 52. 14 periods and 192 - 52 = 140 glyphs remain. These 52 glyphs probalby correspond to 26 weeks (one glyph for the night part of the week and one for the daytime part, i.e. 2 glyphs per week). Summer extends for 7 * 26 = 182 days, half a year. 140 glyphs therefore ought to represent the other half of the year. But 140 / 2 = 70 weeks cannot be correct. The carver of the text must have tried to use the total surface of the tablet (and not stop after 2 * 52 = 104 glyphs). Possibly he created a little riddle: 182 + 140 / 2 = 252 can be read as '2nd 52'. The summer half of the year need not have the same number of glyphs as the equally long winter half of the year.

To secure the numbers I have constructed a table:

16 red glyphs on side a and 36 on side b, a total of 52 glyphs.

Line a3 has an odd number of glyphs (21) and so does line b4 (19). Presumably this is to draw our attention.

The 2nd Rei (which initiates the 1st of the 29 periods of the calendar) has number Ka3-15. The last glyph in the 29th period has number Kb5-14. By using them it is possible to blackmark the number of glyphs which are outside the 29 periods. The sum of the black numbers is (24 + 22 + 14) + (5 + 20) = 60 + 25 = 85. The middle 22 has power to convert 24 into 46  and 14 into 36.

The number of the blue glyphs must then be 192 - 52 - 85 = 55 (= 7 + 14 + 4 + 16 + 14). Both 85 and 55 are numbers which can be divided by 5, hinting that 140 = 5 * 28.

We have had difficulties trying to establish the whereabouts of summer solstice in the calendar of K. Maybe it is easier in Tahua? Comparing the internal parallel glyph sequences, including several promising glyphs, Ab7-36 is an obvious candidate for winter solstice:

Ab7-33	Ab7-34	Ab7-35	Ab7-36	Ab7-37	Ab7-38	Ab7-39

But in the middle of summer there is no such glyph (of course):

Aa5-14	Aa5-15	Aa5-16	Aa5-17	Aa5-18

Instead, the measurement presumably should use the tara glyphs:

(48 + 84) + (90 + 85) + (76 + 82 + 16) = 132 + 175 + 174 = 481. But 1,334 - 481 = 853, not at all the same number. 132 = 6 * 22. 175 = 7 * 25. 174 = 6 * 29.

Noticing how Aa5-14 includes the straight vertical line which in winter is located inside tao in Ab7-38, the measurement maybe should be done by using these markers instead:

481 - 2 (changing Ab7-36 to Ab7-38) - 3 (changing Aa5-17 to Aa5-14) = 476 and 1,334 - 476 = 858 = 13 * 66.

Not very promising. 13 * 36 = 468 would have been better.

Metoro said vero at Ab7-38, and possibly we should measure from there to the special tara in Aa5-17?

481 - 2 (changing from Ab7-36 to Ab7-38) = 479.

Not good. Possibly 3 * 159 = 477 would be OK? Using long count we can reach that number, also if we use short count (but not with normal count):

	480	481	482		851	853	852
	475	476	477		859	858	857
	477	478	479		857	856	855

855 = 19 * 45 is not so bad.

The glyph constellation seems to be a pairwise construction:

Ab7-36	Ab7-37
Ab7-38	Ab7-39

Maybe the 4 glyphs represent the difference between 364 and 360? If so, then Aa5-18 could be the missing 365 - 364.

	482	483	484			851	850	849
Ab7-35				Aa5-17	Aa5-18				Ab7-35