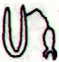|
TRANSLATIONS
We continue with the pages of the 'Excursion':
300 days in the center of the year leaves 60 days to the dark season, with presumably half of it before 'midnight' (winter solstice or the calendar 'crossing over point') and the other half on the 'other side'. I read in Gates about his ridiculing someone named Förstemann - in a detailed example about his complicated constructs in order to understand the Maya texts - and there, suddenly, a little piece to illuminate the 'night': "... This connects up the present section with the beginning of the 'sacred tonalamatl', at the Spring equinox with the Mayas as with the Mexicans, and in the center of the 364-day year (52 days of which preceded and 52 followed the tonalamatl or tzolkin), ruled by its 91-day quarters by the Four Bacabs, whose quarternary repetition (in the 1820-day period) we have thus verified, by discovering what the Maya writer 'clearly' meant to set down ..." In other words: 260 days in the center flanked by 52 days before and after (together 104 days for the 'night'), 260 days beginning at spring equinox. The main structure is a central long season flanked by two shorter ones before and after. Such a structure will survive changes in the details, e.g. moving from 260 to 150 days (or 300). 104 in the 'moon mauga' can be referring to the 'night'.
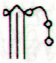
186 = 2 * 93 and in the center 180. Or 186 = 6 * 31 and in the center 6 * 30. These two great seasons (186 respectively 180) can be mapped in the same text, overlapping. 180 will then end with Eb2-7 (see below) and 186 with Eb2-13. There is an ariki two glyphs earlier:
The ariki may be marking the 1st half is in the past - he has 3 feathers at the back of his head, which reminds me about other cases with 3 feathers at the front, e.g. the ariki immediately after vaha mea at the beginning of the text in G:
In Ga1-6 - we note en passent - the sign of 'eating' connected to a 'mauga-fish' seems to generate a season of ragi, beginning at Ga1-6. This season is a consequence, presumably, of the vaha mea and the ariki with 3 feathers in front. We can compare Ga1-6 with Eb2-13. Both glyphs have mauga and the 'eating' gesture. Instead of 3 feathers in front (the first season of the sun), though, there are 4 feathers behind (the last season of the sun). Clearly feathers at the front may mean 'fire' (sun) in front, it will become lighter, while its opposite with feathers at the back (tu'a) ought to mean 'fire' (sun) at the back - it is growing darker (or even - the 'fire' is finished). The position as number 5 may be an indication of the role of the ariki - to engender 'light', giving order. Quite interesting is another ariki glyph somewhat earlier in the 1st period of the E calendar:
He has 4 feathers in front, maybe because he represents the 2nd half of the year (3 feathers for the 1st half year and 4 for the 2nd). The location at 'station 26' implies he is the current ruler. Calculating his location among the 186 glyphs we find him standing beyond position 174 (= 6 * 29), i.e. maybe he is no longer visible. On the other hand, sun is governed by 19, not 29. The numbering nicely defines the last 4 glyphs (Eb2-10--13) as those extracalendrical dark nights which were concluded from the ordinal numbers 2-13. And then - we immediately find - Eb2-8--9 will refer to the 2 nights beyond the 180 which are found by dividing 360 by two (or by counting 6 * 30). The link 'extracalendrical' leads to:
Juggling with numbers and juggling with glyphs. Logic tells me the implied conclusion from elbow ornament in Eb2-10 and Eb2-13 equally well could be that they belong together 'vertically':
Hands held high (Eb2-8 and Eb2-10) are now indicating (in the same way as the elbow ornaments earlier) a group of glyphs which belong together. Eb2-11 will then, however, be in the 'wrong' column (no longer together with the vertical group Eb2-5 and Eb2-9). Another possible glyph order could be:
Now Eb2-11 is once again in vertically correct position. All three horizontal groups are ending with hands held high (the bottom line with Eb2-12 and Eb2-13 together forming the gesture). By moving Eb2-8 upwards one line I felt it necessary to change also its ordinal number from 181 to 180 (leaving only 5 glyphs in the two last lines of my groups). As a consequence Eb2-14 will carry number 6. Another consequence is that all earlier ordinal numbers (for Eb2-7 and earlier glyphs) also must be reduced by 1. This can be done in different ways, e.g. by beginning one glyph later, viz. at Eb1-38:
Or it can be accomplished by not counting the 3 mauga-glyphs and then start 2 glyphs earlier:
This solution is beautiful (and therefore carries power of persuasion): 6-18 at the very beginning (together with Metoro's crossing over - hakapeka), Eb2-1 with ordinal number 6 (because it clearly is an important sun end glyph). The glyph pattern gives a) 180 as the number of days in half a year, b) 182 as 2 * 91 (two quarters), and c) 185 as 365 - 180. Even if this last solution is the one we would prefer to imagine the creator intended us to see, that does not exclude the other readings. Before the logic of excluding took hold of our minds there was a logic of including. To live in a secure world it is necessary to try to assimilate (understand) all facts of life. To see the common traits instead of to see what is different (and therefore strange) was the right way of thinking.
|
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||