The assumed 5-fold division of line Aa1 may result in a possible divsion of the year into quarters:

However, the appearance of Aa1-18 and Aa1-36 in the calendar of the daylight interferes with the interpretation. Aa1-18 is located at 18 / 90 (equal to 20 %) of the way around the guessed at yearly cycle, while at the same time meaning (in the daylight calendar) that sun has appeared at the horizon in the east.

20 % * 360 = 72 days (not 90 days)

Aa1-36 is located at 36 / 90 (equal to 40 %) of the way around, while at the same time meaning that sun has 'died' but reappeared in the form of ahiahi (the fires alighted by the people in the early evening).

40 % * 360 = 144 days (not 180 days)

Therefore there seems to be a double meaning in the glyphs Aa1-18 and Aa1-36. Seen isolated from the remaining glyphs of the day calendar, however, they give the right images for the 5-fold division (quarters + a final 5th solstice period).

With 360 divided into quarters (not 5 parts), 25 % (respectively 50 %) will give the wished for results:

25 % * 360 = 90 days (spring equinox) and 50 % * 360 = 180 days (summer solstice)

25 % = 5 / 4 * 20 % = 125 % * 20 % and 50 % = 5 / 4 * 40 % = 125 % * 40 %

Dividing the year into 5 parts would be natural for somebody who used his fingers as a ‘handy’ calculating ‘machine’.

Furthermore, the terrible Venus gave 5 as her measure:

... only 5 of the 12 constellations in the zodiac are visited by Venus (Taurus, Sagittarius, Cancer, Aquarius, Virgo) ...

... After five complete cycles totaling 2,920 days, the movement of Venus fill eight idealized years of 365 days each and come within hours of spanning 99 lunations ...

 

Dividing a circle into five equal parts is much more difficult, though, than dividing it into quarters. The year evidently has two halves and that is a fact which must govern the structure. The year has to be divided into two halves and there must be a winter solstice addition:

Here we must note that the new year period with 5 dark nights defines the new astronomical year, a year which has two halves defined by the solstices. The 1st half ends at summer solstice and that is evident from the picture in Aa1-53.

The 5 marks on Aa1-72 may refer to the 5 dark winter solstice nights, but at the same time the 5 marks may refer to the 5th part with 18 glyphs (from Aa1-72 to Aa1-90). Double references probably was the rule rather than exception in the rongorongo texts.

On the other hand, another new year was traditionally regarded to begin when summer ended, at the reappearance of the Pleiades around autumn equinox. That year is the one which seems to begin (oho) at Aa1-54. I will call this year for the agricultural year.

Also the ancient Peruvians had two such different years:

"... Garcilasso referred to the ceremonial calendar, which began with the December solstice and after the five extra days which, as in Hawaii, ended the ceremonial calendar at that time; whereas Molina very probably referred to an agricultural calendar, which began in May with the annual appearance of the Pleiades.

This is the more probable since the Inca also regarded the Pleiades as the special patrons of agriculture and marked certain agricultural season by them, while at the same time the pre-Inca Chimu down on the Pacific coast simply considered the rising of the Pleiades as the beginning of their calendar year." (Heyerdahl 6)

Once upon a time, a long time ago, the reappearance of the Pleiades on the horizon coincided with autumn equinox, and at that time the appearance of the Pleiades natually would have been associated with autumn equinox. Later the Pleiades drifted away due to the precession and did no longer serve as an exact mark for new year. The Pleiades furthermore reappeared at different times depending on latitude:

  "... all the principal groups of Polynesia happened to make use of the movements of the same constellation as their chosen sign for commencing the sidereal cycle on which they based their calendar year, namely the Pleiades, when these appear on the horizon in May or June according to the local latitude ..." (Heyerdahl 6)

I think the difference in time due to latitude was a problem which was solved by creating the astronomical year. Solstices and equinoxes happen at the same time all over the globe. The beginning of the astronomical year is similar to Greenwich Mean Time, a longitude zero.

Our next step should be to try to include the lines on side a (with the exception of line a1) into the structure. I will do so by choosing 4 interesting glyphs from each line:

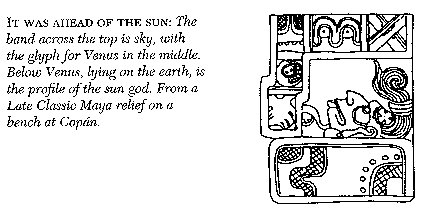
Here much could be said, but we need to concentrate on the main points:

First of all, the new table above is just a preliminary picture. The summer part of the yearly journey of the sun is located at the centre of the text, while winter is divided so that one part appears before summer and the other after summer. Summer is one, while winter is two.

The central part of the human body is the belly. The 'belly of the sun' means the central part of the path of the sun.

... For the Marquesas are given: - daybreak, twilight, dawn, ('the day or the red sky, the fleeing night'), broad day - bright day from full morning to about ten o'clock -, noon ('belly of the sun'), afternoon ('back part of the sun'), evening ('fire-fire', the same expression as in Hawaii, i.e. the time to light the fires on the mountains or the kitchen fire for supper) ...

... When a Central Australian Aranda youngster is between ten and twelve years old ... he and the other members of this age group are taken by the men of the village and tossed several times into the air, while the women, dancing around the company, wave their arms and shout. Each boy then is painted on his chest and back with simple designs by a man related to the social group from which his wife must come, and as they paint the patterns the men sing: 'May he reach to the stomach of the sky, may he grow up to the stomach of the sky, may he go right into the stomach of the sky ...

Winter is split up in two parts and we remember the Y staff in the left hand of the sun god:

... The twelve months of the tropical year (chhi, a very meteorological conception) were divided into twenty-four fortnights, of which twelve were 'chhi-centres' (chung chhi) and twelve were 'chhi-nodes' (chieh chhi - the analogy being with the nodes of a bamboo) ...

In Babylonia they told that Marduk had vanquished Tiamat (the ocean) by cutting through her in the middle:

... Marduk, die Frühsonne des Tages und des Jahres, wurde eben wegen dieses seines Charakters der Lichtbringer am Weltmorgen. Marduk, der die leblose, chaotische Nacht, die keine Gestaltungen erkennen lässt, besiegt, der den Winter mit seinem Wasserfluten, den Feind des Naturlebens, überwindet, wurde der Schöpfer des Lebens und der Bewegung, der Ordner des Regellosen, der Gestalter des Unförmlichen am Weltmorgen ...

... Die Sonne, die des Morgens das Weltmeer durchschreitet und besiegt und das Licht bringt, lässt aus dem Chaos der Nacht zuerst den Himmel, dann erst die Erde hervortreten, spaltet das gestaltlose Reich der Nacht in die zwei Hälften, den Himmel und die Erde ...

I guess that means we should consider the midnight division between the 'days' as the slashing of Tiamat into two halves (the cut being like a node dividing a 'chhi-centre') ...

The structure of the solar year resembles the structure of the diurnal cycle. At 'midnight' there is an illuminated sun sign at the division it two (Aa1-43):

Though the night is yet one and the same, because otherwise the glyph would have been illustrated like the double periods of the day, e.g. (Ha6-10):

Secondly, there are 8 lines but line a1 seems to function as a 'bringer of light' in the middle of the winter, i.e. at the beginning of the 2nd half of winter. Line a1 is similar to Aa1-43. This explains why line a1 contains so much 'sun-light', not only the calendar for the diurnal cycle of the sun but also the primary giver of order, viz. the yearly cycle of the sun as visualized beginning with Aa1-1.

We may even point to the fact that it is Sunday which starts the weekly calendar. After Sunday follows Monday and the domain of the moon is the night. Before Sunday we have Saturday, another dark day. I suggest that the structure of the week must follow the general structure governed by the yearly cycle of the sun.

In Saturday the 'swollen' (ahu) leg at Hb9-52 and Pb10-56 may have been used in rebus fashion to suggest the burial mound (ahu) - like an elevated bump on mother earth:

  

Another 'death' day is Thursday. To explain this the yearly path of the sun functions as a guide. Because we can calculate:

5 / 7 * 364 = 260

Thursday is the 5th day of the week and its position therefore is equivalent to the 260th day of the year.

260 = 13 * 20 while 364 = 13 * 28

13, the unlucky number, announces 'death' on both occasions. The astronomical 2nd half-year must 'die' after 13 moon cycles, and the 'birth' of a new year must coincide with the 'death' of the old agricultural year after 13 double 'hands and feet' counted from wintersolstice.

While 28 results from observing how many nights in a row the moon is shining, number 20 is the result of observing the number of fingers and toes. The shining moon nights cannot, though, be counted up to 28 because counting phenomena they must be of the same kind; the waxing moon nights are different in kind from the waning moon nights. Therefore 28 must be twice 14.

The 'twin' pattern established from counting shining moon nights probably convinced early man to count 'twins' of tens too.

Consequently: 260 = (2 * 10) * 13 and 364 = (2 * 14) * 13.

... Meshing with the 260-day count is a 'Vague Year' or Haab of 365 days, so called because the actual length of the solar year is about a quarter-day more, a circumstance that leads us to intercalate one day every four years to keep our calendar in march with the sun. Although the Maya were perfectily aware that the Haab was shorter than the tropical year, they did not change the calendar accordingly. Within the Haab, there were 18 named 'months' of 20 days each, with a much-dreaded interval of 5 unlucky days added at the end ...

At number 13, Oxlahun, we find the first 'death sign' in the form of a jawbone without flesh.