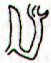To regard the 1st Rei (Ka2-10) as the natural beginning of the following events (which is what I - in the glyph dictionary - have stated is the role of a Rei, leads to recounting, and the results are presented in an additional (2nd and final) page from the link 'beginning':

This little change of the role of Ka2-10 leads to the following reasonable adjustments in our overview table: 1st calendar: 9 A Ka2-1--6 6 9 B Ka2-7--9 3 1 B Ka2-10 1 26 24 C Ka2-11--16 6 12 25 D Ka2-17--22 6 E Ka3-1--4 4 12 13 F Ka3-5--8 4 G Ka3-9--12 4 1 H Ka3-13 1 2nd calendar: 1 0 Ka3-14 1 *74 1 1 Ka3-15 1 20 1-5 Ka3-16--Ka4-12 18 20 6 Ka4-13--14 2 *52 6-16 Ka4-15--Kb1-10 26 *52 16-20 Kb1-11--*Kb2-14 *26 1 21 *Kb2-15 1 I have, though, also divided the disputable period H = 0 in the middle: Ka3-9 Ka3-10 Ka3-11 Ka3-12 Ka3-13 Ka3-14 Ka3-15 G H 0 1 'Ignition' does not occur until Ka3-14, I think (judging from how the glyph is designed). And 3.14 is a well-known number useful for indicating half-year cycles. The number of glyphs in the 1st calendar will then be 26 (as in the 1st and the 2nd halves of 'summer'). Both calendars will begin and end with a single glyph.

26 again. If we count from Ka2-11 up to (but not including) Ka3-15 we get 26 too. In a way that may seem to be the correct way of counting:

Not counting Ka2-10 results in readjusting the glyph into the position of last glyphs among 10. Furthermore, Ka3-14 should be returned into being the last glyph of the 1st calendar, otherwise there will not be 26 glyphs following the first 10. From the presented logic follows that it is not Ka3-14 which should initiate next series of events - it must be Ka3-15 (a Rei glyph).

10 + 26 = 36 in the 1st calendar. Shouldn't the existence of twice 26 in the 2nd calendar make us search for twice 10 immediately before those 52?

There are 20 + *52 = *72 glyphs beyond Ka3-15 (not including the Rei this time). In order to include Ka3-15 we must eliminate 1 glyph of those between Ka3-15 and *Kb2-15.

*52 is a consequence of *26 for the sequence Kb1-11 -- *Kb2-14. Only in this interval can a reduction by 1 glyph be acceptable. As a consequence we must then add Kb1-10 to the new sequence - otherwise the glyphs will not add up to 26. But this operation would once again result in a non-Rei glyph in the first position:

Kb1-10	Kb1-11

A bird's eye view is needed. Some of the fundamental facts of the table presented in the glyph dictionary can be simplified to the following table:

There is no asterisk indicating reconstructed numbers. We immediately can discern a new pattern which resoves the difficulties:

9		26		20	26
	Ka2-10		Ka3-15			Kb1-11
10		27			27
84

84 is a kind of confirmation. 84 = 10 + 27 + 20 + 27. There are 84 glyphs from the beginning of line Ka2 up to and including the 3rd Rei. As a consequence it seems necessary to restart counting from Kb1-12:

1	2	3

To reach 26 once again, *Kb2-15 must be included as the last glyph:

Ka4-13	Ka4-14	Ka4-15
46	47	48

25					*22
	Kb1-11	Kb1-12	Kb1-13	Kb1-14
	0	1	2	3
	74	75	76	77
52

*Kb2-15	*Kb2-16	*Kb2-17
*26	*27	*28
*100	*101	*102