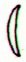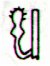|
TRANSLATIONS
The tide pattern is slowly moving: '... Its period is about 12 hours and 25.2 minutes, exactly half a tidal lunar day, the average time separating one lunar zenith from the next, and thus the time required for the Earth to rotate once relative to the Moon ...' The tidal lunar day is 2 * 12 = 24 hours + 2 * 25.2 = 50.4 minutes long. How long is the next greater cycle, a tidal lunar 'month'? The offset from the diurnal cycle must be those 50.4 minutes per day. If we assume the tidal lunar 'month to have two equal parts ('fortnights', resembling the pattern of waxing and waning as observed in the month and year), we must let those 50.4 minutes rotate twice. There are 24 * 60 = 1,440 minutes in a day. There is room for 28 times 50.4 minutes in a day. 28 * 50.4 = 1,411.2 minutes, leaving a residue of 1,440 - 1,411.2 = 28.8 minutes (ca 29 minutes). 28 often appears in the rongorongo calendars, and I have up to now considered it as due to counting the nights when moon is visible (from the light of the sun). The moving tide pattern cycles around twice in ca 28 days. When e.g. full moon is straight above at midnight, i.e. coincides with high tide, next similar occasion will come 28 nights later. In between a new moon straight above will coincide with another high tide. However, when 28 nights have passed, the tide pattern difference (50.4 minutes extra needed for each day) has accumulated to ca 1 day, i.e. it needs 29 nights (not 28) to complete its cycle. 28 (50.4 + 24 * 60) = 41,731.2 minutes = 695.52 hours = 28.98 days = ca 29 days The synodic month is ca 29½ nights, not ca 29 nights. The difference, ca ½ níght, results in 7 days in a year.
The full moon (and all the other 'phaces' of the moon) cycles because of the diurnal rotation of the earth. When moon, sun and earth are located in a line, new moon is the situation with moon between earth and sun while full moon is when earth is between moon and sun. In the latter case full moon is visible straight ahead at midnight. During a year one more rotation than the diurnal must be considered, the rotation of the earth around the sun. The synodic month therefore differs from the sidereal month: 27.3 = 29.5 - 29.5/365.25 * 27.3 29.53 - 28.98 = 0.55 must be due to the rotation of the moon around the earth. 24 * 60 + 50.4 = 1490.4 29.53 / 1490.4 * 28.98 = 0.57 = ca 29.53 - 28.98 (= 0.55) High tide (respectively low tide) occurs twice a day. Measuring 'seasons' of high tide and low tide, we will have 4 seasons in a day. We recognize the quarters in a year. There are 4 corners for the earth. Low tide should correspond to the time when the sand is exposed to the rays of the sun. We remember the flounder:
We ought to count in the Mamari moon calendar. First I find the ordinal number of Ca7-24 (Omotohi), counted from the first glyph in the calendar (Ca6-17) to be 36. Relying on the numbers, we can then confidently eliminate Ca9-1--2 from the calendar:
The number of glyphs for waxing moon must be equal to those of waning moon. Twice 36 is 72. Furthermore, a new line of glyphs (a9) begins at Ca9-1. The text starting with Ca9-1 is probably 20 glyphs long (twice 4+6 glyphs):
We notice how two marama glyphs (red-marked) initiate the first decade, after which the next decade has only one marama and this one reversed. I think this is the opposite pattern to what we can read in the rongorongo texts about the solar year - it begins with 1 and takes number 2 at the beginning of the 2nd half of the year. Cfr Aa4-58 and Aa4-60. Ca9-9 (koti) has twice number 9, a coincidence? At the beginning of the Mamari moon calendar we must similarly organize the glyphs to find out if we really have assembled all glyphs belonging to the calendar. We should begin already at Ca5-20:
Another sequence of 20 glyphs then follows (with the pattern 7+3 and 4+6 glyphs):
Without doubt the moon calendar begins at the proper place, at Ca6-17: Ordinal number 16 in Ca6-16 ends the 20-group above. Ordinal number 16 in Ca7-16 ends the 3rd period of the moon calendar, while ordinal number 16 in Ca8-16 is marked by curious wings:
A table showing the ordinal number 16 glyphs. The 'hua' stage implies 'end':
|
||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||