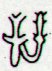|
TRANSLATIONS
If we keep the model just arrived at in our heads and start to read at the beginning of the night time in the H calendar we see:
Ha5-37 has now changed its role. Following the lead of the P calendar (as we see it for the moment) this is not only the beginning of po ('night' = 'day') and the beginning of the dark part of po (as opposite to the sunlit light part of po), but Ha5-37 also stands for the beginning of the lunar (sun-lit) period, The sun-lit lunar period consists of 28 nights. The beginning comes immediately after new moon (according to the Mamari moon calendar - and my reading of that calendar), i.e. Ha5-37 stands at the position of Ohiro. "...when the new moon appeared women assembled and bewailed those who had died since the last one, uttering the following lament: 'Alas! O moon! Thou has returned to life, but our departed beloved ones have not. Thou has bathed in the waiora a Tane, and had thy life renewed, but there is no fount to restore life to our departed ones. Alas'..." (Makemson) Yet there is a kind of reassurance here: When sun goes down and darkness is threatening the moon is rising (according to my current reading of the po calendar), and the moon has been revitalized in the living waters of Tane (or whatever he was called on Rapa Nui), she is shining again illuminating the night. We may imagine that this was how the P calendar should be read. Whether the H calendar has a similar or different reading is not yet clear. But there are only 12 glyphs during its night part. To reach full moon from Ha5-37 we need to take some glyphs from the day part. According to my reading of the Mamari moon calendar have this situation:
To reach full moon (Ca7-24) 15 nights is needed. 15 - 12 = 3, which means that Ina-ira, Rakau and Omotohi must be in the light part of the H calendar of po (if there is similar kind of moon calendar intertwined with the 'day' calendar). Obviously this means (which we also found in the P calendar) that the 1st period of daylight in a way belongs to the night:
I have not only once used the word 'intertwined' to describe the double 'faces' which are visible in the calendars. To my surprise, when I searched for the meaning of the word Ohiro I found that it had a similar meaning:
The moon indeed rises from the waters by itself and a little knowledge of astronomy tells us that it is spinning on itself. Although the moon always turns her back away from us; her back is unknown. Easily it would have been understood, in very ancient times, that the moon spins on her axis, because the frame of reference was the starry heaven. The moon moves against the background of the fixed stars and if we never can see her back, then she must be slowly revolving on her axis, keeping her back against the stars. It is interesting to see that the number of glyphs in the 2nd part (of the periods) in the Mamari moon calendar is 36, a clear reference to the 360 'days' in a solar (calendrical) year. Not only do we find that the 'day' calendars seem to be intertwined with the lunar cycle, but also that the moon calendar has signs referring to the solar cycle. Also we remember that in Hawaii the solar cycle was intertwined with the full moon (and the Pleiades): '... The correspondence between the winter solstice and the kali'i rite of the Makahiki is arrived at as follows: ideally, the second ceremony of 'breaking the coconut', when the priests assemble at the temple to spot the rising of the Pleiades, coincides with the full moon (Hua tapu) of the twelfth lunar month (Welehu) ...' (Islands of History) We may now possibly redefine the structure of the H calendar into this:
But maybe the night should have 12 periods, which is possible if we transfer the last daylight period to the night (in a way similar to the proposed transfer of the first daylight period to the night). If we do that, however, the number of periods for the daylight part of the calendar (which initially was perceived as 9) will be reduced to 7 (equally unacceptable as 9 because of being odd numbers). If we refer the X part of the calendar to the night and regard that part as a period, then night will have 12 periods (10 + dawn + X) and daylight time will have 8 (9 minus dawn), with 20 still as the sum of all periods. 12 (night) reflects sun and 8 (day) reflects moon. In H, P and Q we have X following night, at the end of the calendar, whereas in A it is X that initiates the calendar. Adding X to night in H, P and Q seems easy, whereas in A it is more natural to assume a relationship between X and day. Recounting the glyphs according to the proposed new grouping of the periods leads to 12 + 3 + 8? = 23? for the night and 8 + 8 + 13 (?) = 29 (?) for the daylight time. (Maybe this means that we should reconsider and refer 14 glyphs to p.m. and 7 to X, by way of which we reach the more reasonable sums 22 and 30?) We do not have the facts and we do not know. But let's anyhow see where it all leads, by rewriting the total table:
In H, P and Q we can now read 28 and 29, presumably signifying number of moon nights. There is a question mark in H, but question marks do not deny. In Q 29 may be read as 28 + a fraction (because Qa5-55 is just part of a glyph). In A there is no sign of 28 or 29. If we add the 2 dawn glyphs to night we get 14 glyphs for the night. Adding also the 2 glyphs for the last daylight period makes the sum 16. Adding also the 3 X glyphs makes the sum 19. Dawn + X (and not adding the two glyphs in the last daylight period) makes the sum into 17. Obviously we should not try to add the X glyphs to the night. We must regard the A calendar as totally different from the other three calendars. In H we must reconsider again. Not finding any support (as in P and Q) for the number of sunlit moon nights (28) we should instead look at A. Maybe (after having transposed 3 glyphs in dawn to the night) we have 30 glyphs for daylight time, i.e. we need 4 glyphs (instead of 3) in the last daylight period:
Ha6-19 maybe once had a twin at right? That seems probable and we then arrive at the more satisfactory 30 as the number of glyphs after dawn and up until nightfall. Adding a glyph like this, however, increases the total number of glyphs for the calendar from 52 to 53, which cannot be right. Therefore we decrease the X glyphs from 8 to 7. After recalculating and revising the structure of H to be like that in A we may then once again rewrite the total table:
We must put a stop here and leave (for the moment) the question about how structures and numbers intertwine. |
||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||