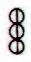TRANSLATIONS

next page previous page up home

Now I have convinced myself about the central importance of Saturday, and especially then the GD65 glyphs (Hb9-42 and Pb10-47):

  

A suspicion immediately arrives: What ordinal numbers do they have in the weekly calendars?

Hb9-42 (!) is number 26 (and there are 42 glyphs in all in the calender according to H).

26 * 14 = 364 and 16 (i.e. 42 - 26) * 14 = 224 = 364 - 140 = 8 * 28.

42 = 26 + 16, with Sunday having 5 glyphs and Thursday beyond Hb9-42 also having 5 glyphs. Five means fire I am certain of - as the number of fingers on a hand.

Monday has 8 glyphs (making us think about the Mamari moon calendar with 8 periods). Saturday has also 8 glyphs, while we have to add the 3 glyphs of Friday to those 5 'fiery' ones after Hb9-42 to reach 8.

Tuesday has 3 glyphs too, but adding Wednesdays 6 glyphs we reach the ominous sum 9, and darkness is also what we can read in Hb9-38:

A black-marked oval may express that the fire (of the sun - 6 glyphs in Wednesday) is put out before a new one is kindled in Thursday. Water quenches fire and water is the element of Wednesday.

Adding the glyphs after Monday to reach Hb9-42 we get 13, perhaps also an ominous number.

Examining P we first find that with 10 glyphs we have covered Sunday + Monday (while there were 13 in H for the same period).

Adding together Tuesday, Wednesday and Thursday up to (and including Pb10-47) we reach 9 glyphs.

From the beginning of the week and up to (and including) Pb10-45 we reach 17 glyphs:

As there may be glyphs missing at the end of the week according to P it is uncertain how many glyphs there once were in Saturday, but if we put a missing manu rere at the end we get 8 (equal to the number in H).

The total number of glyphs in the P calendar would then amount to 10 (Sunday + Monday) + 24 (the five remaining days).

The five otters trying to steal a fish from the barbecue may be a symbolic picture for the five planets, the moon (the fish) and the sun (the barbecue).

Sun and moon should, though, hardly be counted together in the weekly calendars:

  H P
Sunday 5 26 3 18
Monday 8 7
Tuesday 3 3
Wednesday 6 4
Thursday 'a.m.' 4 1
Thursday '´p.m.' 5 16 5 16?
Friday 3 3
Saturday 8 8?
number of glyphs, sum: 42 34?

Here I have tampered with the location of GD65: I have counted Hb9-42 to 'a.m.' but Pb10-47 to 'p.m.'

Not only do we thereby reach 16 in both tablets but there are no 'a.m.' glyphs in P (more than the one initiating Thursday). However, coming so far it becomes rather obvious that the structure in P should be described instead like this:

Sunday 3 26
Monday 7
Tuesday 3
Wednesday 4
Thursday 6
Friday 3
Saturday 8 (?)
number of glyphs, sum: 34 (?)

26 is the fundamental number (which also H exhibits). Not only are there 26 * 14 = 364 days in a year, but 26 seems to have been used as a basic number which other interesting numbers were combined with:

26 + 10 = 36

26 + 16 = 42

26 + 8 = 34

The 8 (?) glyphs of Saturday tell us that Saturday is outside the 26-glyph main group of glyphs. Saturday is a day of temporary death. Temporary death (caused by the kava ceremony) may also be the reason why the H text has 26 as a group ending in the midst of Thursday.

Reexamaning the beginning of the Tahua text (on side a) we immediately then understand that 26 appears in Aa1-26 as the last glyph of a.m.:

That we knew already, because of the open hakaturu 'tail' at right, because the Q text ends at this point, because tapa mea is reversed in Aa1-27, because sun is 'death-marked' by the sign of Y, and because the tapa-beater (ike) is hitting him at Poike (noon).

By adding 16 to this 26 we reach Aa1-42, which we now understand is the last glyph of the 1st half of the night period. Thereby we define 6 glyphs for the 1st half of the night and 6 glyphs for the 2nd half of the night.

Number 42 makes me remember an idea I had some time ago: Consider that the last line on side b in Tahua has 84 glyphs. Consider, furthermore, that 42 and 36 are important signs as indicating 'outside' the main text:

Side a has 670 = 200 * π + 42 glyphs and side b has 664 = 200 * π + 36 glyphs; Aa1-36 is the last glyph in the daylight calendar while Ab8-43 marks the 'crossing over place':

We then recapitulate what I once wrote about the triple rhomb type of glyph:

Ab6-88:

Here the 'balls' are changed into rhombs, meaning three lunar double-months. Wheareas the solar double-months have 2 * 30 = 60 days, the lunar double-months have 30 + 29 = 59 nights.

There is no vertical straight line in the middle of the glyph. 59 is an odd number and cannot be divided into twins. Also there is no measurement, the moon is a 'clock' in itself.

The four corners in the rhomb marks where periods of 14 nights are completed. 4 * 14 = 56 = the number of nights in a two-month period when the moon is visible.

In a rhomb the 14 nights when the moon is waxing may be seen as the upwards going line at left, the apex being the time of full moon. The waning moon will then be represented by the downwards sloping line at right. At the time of new moon we turn the rhomb 180º similar to when we start to read a rongorongo tablet (though here clockwise instead of counter-clockwise), and after that we can start once again with the waxing moon of the new month.

Moon and sun are complementary. And the texts on the rongorongo tablets turn counter-clockwise only every odd turn, the even turns are clockwise. Right and left alternate, just like sun and moon. The sun is right, the moon is left.

The counter-clockwise movement seems to be connected with the sun (south of the equator) and the clockwise movement therefore with the moon. Consequently, the waning moon should be the downwards sloping line at right.

"The 'second list of place names' appears for the first time in Ms. E. The sequence of the places named runs opposite to Hau Maka's 'first list of place names'. Commencing on land at a point 'that can send signals' out to sea, the path turns to the right along the coastline so that the ocean is always on the left. On the other hand, proceeding from the land of origin with the description of the route to the new land, Hau Maka's place names are intended to provide a route that starts from the ocean and along which the ocean is always to the right.

We seem to be dealing here with two possible inversions; turning 'toward the sea' vs. turning 'toward the land', while maintaining the same general direction, which is described by the common Polynesian contrast pair tai vs. uta, or turning 'toward the right' vs. turning 'toward the left', facing in the same direction at the start." (Barthel 2)

The 'first list of place names' (sun) seems to have been handed down in writing, the 'second list of place names' (moon) presumably was passed on with kaikai:

"While the 'first list of place names' is supposed to have been handed down explicitly in writing (ta ki runga ki te kaka), the 'second list of place names' was passed on with the aid of a mnemonic device in the form of recitations (patautau) accompanied by the string-figures (kaikai)." (Barthel 2)

Let us reason the other way around: 84 divided by 3 equals 28 (the number of nights in a month when moon is visible). 14 is twice the weekly number, and 28 = 2 * 14. A rhomb may therefore be understood as the period when moon is shining on the earth. Each side of the rhomb marks a week (not a fortnight which I earlier suggested).

Metoro's reaction to this type of glyph (inoino, bad-bad) maybe was because after 84 nights there was needed 6 nights intercalated to reach the sun triple-month period 90 days, a period corresponding to the time for moving from one of the 4 cardinal points (solstices and equinoxes) to the next.

Still the standard GD53 with ovals may continue to mean 6 solar months:

However, another solution is to see 3 solar months and an additional day (the vertical straight line) to reach 91 days. Instead of reading 3 solar double-months we may read 3 single solar months + 1 additional day.

We now reach a kind of symmetry between the two glyph types:

  

They both mean a quarter, but the triplet of rhombs measures 3 * 28 = 84 days, whereas the triplet of balls measures 3 * 30 + 1 = 91 days. The difference is a week (7 days).

We may then imagine a greater rhomb measuring the whole year (364 days). At each corner of this rhomb we have an extra week, while each side of the rhomb measures 84 days.

4 * (84 + 7) = 364.

The solar year, on the other hand, is divided in two halves, with 2 * 91 = 182 days in each half. Such a half could be represented by a double GD53, as e.g. in Aa7-9--10:

Maybe the same sign was used to indicate the Pleiades. One half of the year they are 'above' and the other half of the year they are 'below'.

I think this reading of the glyphs is better than what I suggested earlier.

But then we must reconsider: The reason for 84 glyphs in lines Ab7 and Ab8 may be to express half a year (measured by the lunar weeks). Neither line Aa1 (with 90 glyphs) nor lines Ab7 and Ab8 (with 84 glyphs) express the extra 1 day respectively the extra 1 week.

a1 90 b1 82
a2 85 b2 85
a3 76 b3 77
a4 82 b4 80
a5 83 b5 80
a6 84 b6 92
a7 85 b7 84
a8 85 b8 84
sum 670 sum 664

Furthermore: In addition to 400 * π we have 36 + 42 = (26 + 10) + (26 + 16) = 3 * 26.

Are these 3 comparable to Aa1-13--15?