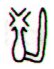I decide to count the glyphs of the P text (not done before) - with numbers of glyphs proving to be so important it seems to be necessary. Besides, if we can get a grip on the structure of P it could help us with using not only the glyphs of P but also those of H and Q.

The result:

The redmarked glyph numbers are included here (but not otherwhere) to show where the rule 'odd line numbers should have an even number of glyphs and the reverse' fails. The creator of the P text seems to have been unaware of this rule (or used a more sofisticated rule prohibiting its use).

599 + 559 = 1158 = 6 * 193 does not strike any bell.

If we add an imaginary 1 on each side, however, we get 600 + 560 = 1160 = 40 * 29. Is that in reality or just in my mind?

Of course we must then count to Pb10-1:

1043 = 7 * 149, not very interesting. But if we add one of those imaginary 1:s, we reach 1044 = 4 * 261.

The other way around we will have 1158 - 1043 = 115 = 5 * 23, not so amusing. Alternatively we add those imaginary 2 and subtract 1044. 1160 - 1044 = 116 = 4 * 29. We have then used one of the imaginary 1:s before Pb10-1 and the other beyond Pb10-1.

As we know 261 = 9 * 29, and therefore the total number of the P glyphs can be thought of as (36 * 29 + 4 * 29)  - 2 = 1158.

Is there more reality in my numbers now?

We must have more 'proof'. There are 3 viri glyphs (without additional signs) in P. One of them is special (Pb9-21), drawn with a more powerful 'body'.:

I have counted backwards from 1158, taken away 50 (b9), 56 (b10) and 60 (b11) and then added 21, 24 respectively 29. Between Pb10-1 and Pb9-29 (with most interesting ordinal numbers) there are 21 glyphs.

Should we try to walk 261 (= 9 * 29) glyphs forward or backwards from Pb9-29? (Or from Pb9-21?).

261 - 28 (in line b9) = 233. Summing backwards from line b8: 65 + 63 + 59 + 50 = 237. Are we looking for Pb5-4?

Pb5-4 indeed shows traits which indicate we are on the right track - a sun with a strange appendix at left ending with a hole.

The appendix with a hole is a sign of the pu glyph type, which makes me remember the last glyph in the G text (Gb8-30), which also is classified - according to my system - as a pu glyph:

There are two 'holes' in Gb8-30, but only one in Pb5-4. Maybe there is a two-hole pu glyph 261 glyphs beyond Pb9-29? (Or somewhere close by, because we have those two imaginary glyphs to bother about too.)

Using the glyph catalogue we can see 18 pu glyphs in P:

261 glyphs ahead of Pb9-29 (9 * 29 = 261): we count 21 (b9) + 56 (b10) + 60 (b11) = 137 and 261 - 137 = 124. Then we add from the beginning of side a: 53 + 59 =112, the searched for glyph should be around Pa3-12:

It was no pu glyph, but perhaps anyhow what we are looking for. 125 = 5 * 5 * 5 is a good number. And we recognize a triplet of surrounding signs similar to for instance Gb3-26:

The 'fists' show how light still has not arrived, but there is promise.

Tentatively we can add Pa3-13 to our hypothetical structure:

Is this a statement of 21 * 25 = 2 * 261 + 3? Moving outside those 525 glyphs there must be another 1158 - 525 = 633 glyphs, an uninteresting number. We need to reduce by 5 to reach 628.

In Tahua we saw how te pito was correlated with viri. We therefore ought to see if that is the case also in P:

Yes, it is so by being close to the three viri glyphs and we should add te pito to our 'building':

Maybe we should count 261 beyond te pito (Pb9-33) and 261 before te pito?

We have arrived at what I earlier called the 'Matariki calendar'. Between te pito and Pb5-8 there are 261 glyphs.

Pb5-19 is interesting because its middle looks like a 'gnomon' type of glyph. Presumably it is the same 'person' (hakaturou) as we see bending down in Pb5-8. The ordinal number of Pb5-19 is 774, or 18 * 43 which maybe implies 'end of summer'. It ought to be related to Pb10-1 (1043). 1043 - 774 = 269, which hardly is meant. 1158 - 269 = 889 is more suggestive - 888 glyphs between Pb10-1 and Pb5-19:

We presumably ought to coordinate Pb10-1 and Pb5-19 with these three glyphs:

Pb5-4 has ordinal number 759. There remain 50 - 4 = 46 glyphs in line b5. 759 + 46 = 805.