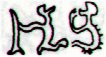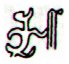TRANSLATIONS

next page previous page up home

We found 12 hau tea on side a and 15 on side b. We will now calculate how many ihe tau there are on each side. This time we will start by showing all GD45 glyphs in Tahua according to the glyph catalogue:

There are altogether 12 * 6 = 72 glyphs on both sides. Although 72 = 2 * 36 the glyphs are not equally divided on the sides, there are 40 on side a and 32 on side b. These numbers (72, 40 and 32) indicate that the creator had a system.

Next we discard all glyphs with additions and the remainder are:

7 * 6 + 2 = 44 glyphs, 25 on side a and 19 on side b.

The numbers 44, 25 and 19 do not seem promising. However, they are not random, which our next step will show.

Obviously the variants of GD45 show some kind of pattern. To make this more clear we will now add the glyph labels and sort the glyphs according to variant:

- Fat, open at bottom left, a hint of a spur at bottom right. 1 6 10
Aa1-12
1   5
Aa1-49 Aa1-50 Aa1-51 Aa3-74 Aa4-3
3 Red labels for marked glyphs. 4
Aa4-48 Aa4-51 Aa4-66 Aa4-75
2 6 10
Aa5-46 Aa5-54 Aa5-57 Aa5-64 Aa5-83 Aa6-41
3 Aa4-75 and Ab2-7 belong to this group. 4
Aa7-19 Aa7-26 Aa7-36 Aa7-48
- A variant in between 3 and 5. 2 6
Aa7-57 Aa7-68
- 10 + 10 + 6 = 26 = 15 + 11 4
Aa7-72 Aa7-81 Aa8-23 Ab1-10
3 The 6th and last glyph of this kind (3). 1 5 18
Ab2-7
4 Open at top. 4
Ab2-31 Ab2-51 Ab2-68 Ab3-3
5 13
Ab4-11 Ab5-12 Ab5-36 Ab5-38 Ab5-40 Ab6-85
Ab7-13 Ab7-18 Ab7-20 Ab8-44 Ab8-66 Ab8-68
Variant 5 has 1 + 12 = 13 glyphs, reminding us about 13 * 28 = 364.
Ab8-78

26 + 18 (= ½*36) = 44.

The GD45 glyphs with additions on them are 72 - 44 = 28 in number:

Aa1-11 Aa3-51 Aa4-20 Aa4-57 Aa4-59 Aa4-62
Aa4-80 Aa4-82 Aa5-20 Aa6-3 Aa6-30 Aa6-55
Aa7-76 Aa8-56 Aa8-60 Ab1-37 Ab3-4 Ab3-65
Ab4-6 Ab4-7 Ab4-9 Ab4-12 Ab5-3 Ab5-13
15 (side a) + 13 (side b) = 28
Ab6-41 Ab7-41 Ab8-70 Ab8-82

Reviewing the hau tea glyphs we similarly can arrange the complex glyphs into a table:

Aa1-28 Aa1-74 Aa5-19 Aa6-2 Aa7-29 Aa8-20
Aa8-54 Ab1-2 Ab1-23 Ab1-24 Ab1-25 Ab2-45
Ab3-16 Ab4-14 Ab4-20 Ab4-25 Ab4-30 Ab4-42
Ab4-47 Ab4-57 Ab4-62 Ab5-24 Ab7-2 Ab7-40
 26 glyphs = 14 + 12 (without 'eyes')
Ab7-59 Ab8-81

12 glyphs which I have only tentatively classified as GD41 (marked red above) form a set which maybe should have a separate GD. The sum 26 is only a weak indicator that I may have done a correct classification. I cite my definition of GD41:

'As GD41 I furthermore have decided to define such glyphs which are curved and without the vertex, as e.g. Ab5-24, Ab4-30, Ab7-59, Aa1-74 and Ab7-2:

           

Presumably they mean something else than the straight-lines-with-vertex glyphs, but they must be located somewhere, and it became GD41.'

Ab7-2, I now guess, belongs to the 'true' GD41 because the 'head' may be regarded as the 'eye' in the normal GD41 (remember manu mata toru as Metoro said somewhere).

If I am wrong now (and was right when I defined GD41), then 26 = 13 + 13 and the 'true' (but complex) GD41 are 6 (side a) + 7 (side b) = 13. The rest (red + Ab7-2) are 1 (side a) + 12 (side b) = 13.

As a last step we incorporate the 28 non-complex hau tea glyphs in the calculations:

GD41 non-complex glyphs complex 'true' other sum
side a 12 6 1 19
side b 15 2 12 29
sum 27 8 13 48

Because of the sum 8 I have come to accept as correct my earlier classification of Ab7-2 as 'other' (than 'true'). Furthermore, 48 indicates that also the 'other' glyphs should be classfied as GD41. 48 is a better sum than 35 (=27 + 8).

GD45 non-complex glyphs marked d:o complex sum
side a 15 10 15 40
side b 12 2 13 27
sum 27 12 28 67