Dots adorn all 5 glyphs at the beginning of line a14. Only the top of the images are visible:
We can compare with the beginning of the text (where day 81 is March 22 because there is no February 29 in the year before a leap year). There is a vero sign in Ca1-1, perhaps because of the equinox:
As I have reconstructed the text there are 29 glyphs in the last line on side a. Beyond the first 5 glyphs there seems to be a triplet:
Then there are 2 more (in addition to that in Ca13-19) vero glyphs. 378 is the synodic cycle of Saturn, but what is 372?
In a leap year April 1 will be day 92 and April 4 day 460. Vero in April 2 is drawn like vero in March 17:
Possibly the months in the lunar year are only half as long as those in the solar year. 458 = 2 * 229. 12 glyphs remain in the line, but I prefer to save them for the next page. 392 - 12 = 380 = 460 - 80. As to my question about 372 the answer maybe can be found in G, because in that text we have to add 64 + 80 = 144 in order to reach the Gregorian day numbers. In C we evidently need to add only 80. 372 + 64 = 436, i.e. Ca14-9 could correspond to Gb7-25 (where 72 * 5 = 360):
If my suggested heliacal dates at the beginning of side a of the G tablet can be extrapolated backwards to cover also the end of side b, then vaha mea in Gb7-25 will correspond to April 17 (107) when in rongorongo times Polaris and Sheratan rose heliacally. To be able to observe these stars when culminating at midnight would necessitate moving ahead ca 233 days to early December:
If, on the other hand we should move ahead from the beginning of side a all the way to the end of side b, then the heliacal date at Gb7-25 will be 436 + 64 = 500 days beyond March 21 and we need to add 80 (or 81?) to find the Gregorian date. 500 + 80 - 365 = 215 (August 3), when Acubens (α Cancri) rose heliacally:
215 + 365 - 472 = 580 - 472 = 108 (and 442 + 365 - 705 = 102). March 27 (at vero in Ca14-9) does not fit with any of the redmarked dates listed above. Another alternative is then to think the nakshatra way to see what stars were visible in the night close to the full moon in March 27. Once again there are 2 alternatives:
26.6 (Polaris) + 365¼ / 2 = 209.2 and 135.0 (Acubens) + 365¼ / 2 = 317.6. There seems to be only one acceptable star candidate, viz. η Ursae Majoris, which was close to Sun at the antipodal point in the sky in July 27 (208).
Polaris (and Sheratan) could be observed in July 27 and Benetnash in Gregorian day 472 - 365 = 107 (April 17). 208 (July 27) - 86 (March 27 in a normal year) = 122 = 2 * 61. 451 (March 27 in a leap year) - 208 (July 27) = 243. At Rau Hei this number was found to be a measure from 'going down' to 'rising again':
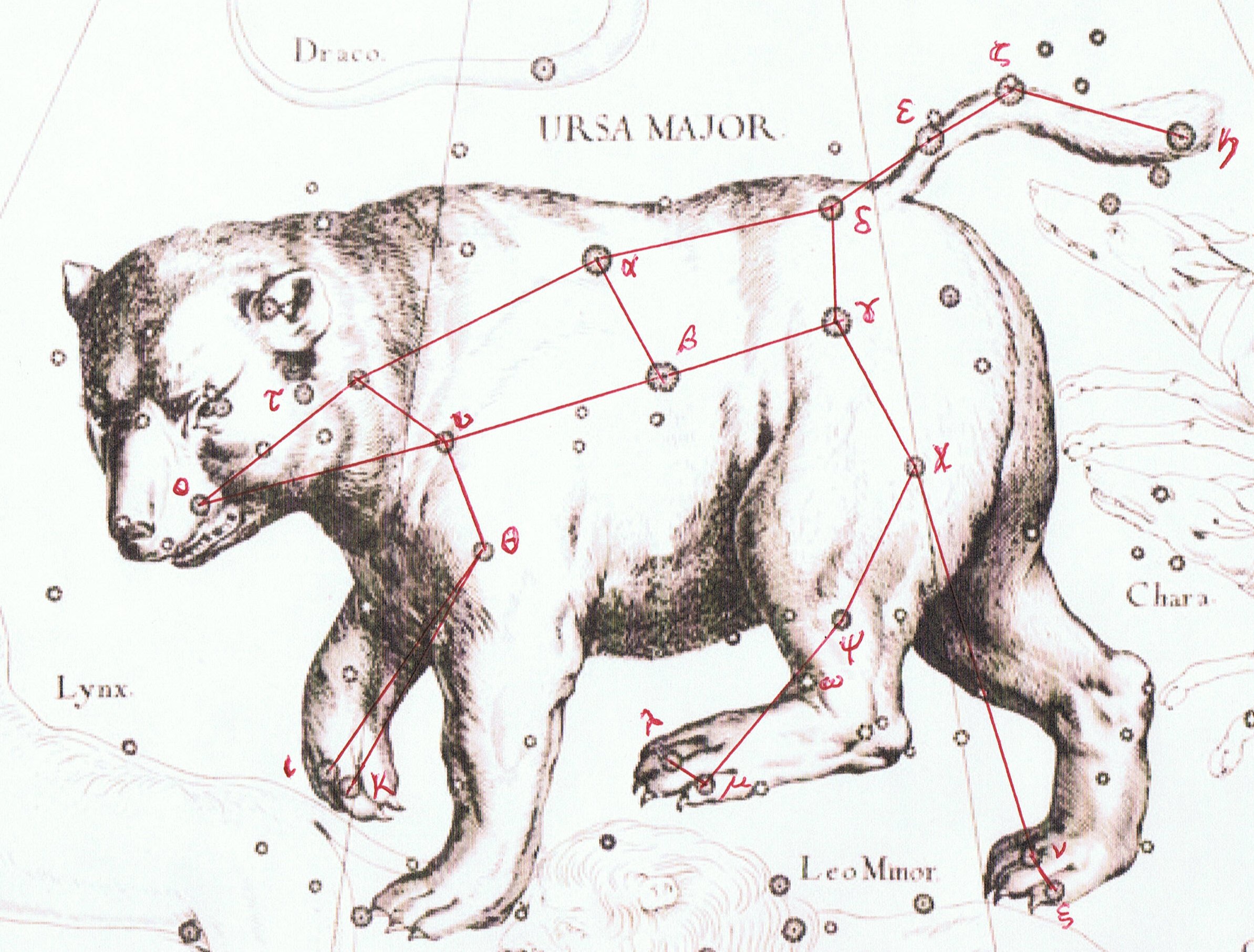
243 + 107 = 350 and 243 + 122 = 365 (a normal year). ... The name [Benetnash] derives from the Arabic phrase meaning 'The leader of the daughters of the bier'. The daughters of the bier, i.e. the mourning maidens, are the three stars of the handle of the Big Dipper, Alkaid, Mizar, and Alioth; while the four stars of the bowl, Megrez, Phecda, Merak, and Dubhe, are the bier ... Possibly these 4 (bier) + 3 (daughters) - to mention them in time order - explains why there was a slave missing when the bier of dead Ceasar was to be carried. Thus, in March 27 the leader of the daughters carrying the bier could be seen close to the full moon. I cannot see any obvious such indication in the pictures in or around Gb7-25. But vero in Ca14-9 surely must indicate a mortal threat to some ruler.
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||