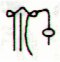
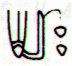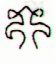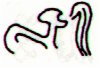Also Ca1-6 could depict the nighttime 'smoking mirror' image of Sun, at least he appears to have a great droplet-formed hole in his chest (the rongorongo idiom does not allow tears to be drawn in the face of a person):
Counting from Ca1-6 to Cb14-17 we find a somewhat reassuring 738 - 6 = 732 = 2 * 366. It could mean there were 8 (= 740 - 732) glyphs outside the solar calendar year. "... According to the later writers Censorinus and Macrobius, the ideal intercalary cycle consisted of ordinary years of 355 days alternating with intercalary years, alternately 377 and 378 days long. On this system, the average Roman year would have had 366╝ days over four years, giving it an average drift of one day per year relative to any solstice or equinox ... In practice, intercalations did not occur systematically ... but were determined by the Pontifices ... They usually occurred every second or third year, but were sometimes omitted for much longer, and occasionally occurred in two consecutive years. If managed correctly this system could have allowed the Roman year to stay roughly aligned to a tropical year. However, since the Pontifices were often politicians, and because a Roman magistrate's term of office corresponded with a calendar year, this power was prone to abuse: a Pontifex could lengthen a year in which he or one of his political allies was in office, or refuse to lengthen one in which his opponents were in power. If too many intercalations were omitted, as happened after the Second Punic War and during the Civil Wars, the calendar would drift out of alignment with the tropical year. Moreover, because intercalations were often determined quite late, the average Roman citizen often did not know the date, particularly if he were some distance from the city. For these reasons, the last years of the pre-Julian calendar were later known as 'years of confusion'. The problems became particularly acute during the years of Julius Caesar's pontificate before the reform, 63 - 46 BC, when there were only five intercalary months (instead of eight), none of which were during the five Roman years before 46 BC. Caesar's reform was intended to solve this problem permanently, by creating a calendar that remained aligned to the sun without any human intervention. This proved useful very soon after the new calendar came into effect. Varro used it in 37 BC to fix calendar dates for the start of the four seasons, which would have been impossible only 8 years earlier ..." (Wikipedia) | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||