2. However, my culmination table can be misleading because it counts the difference in the dates in the wrong direction, going back in time instead of forward.

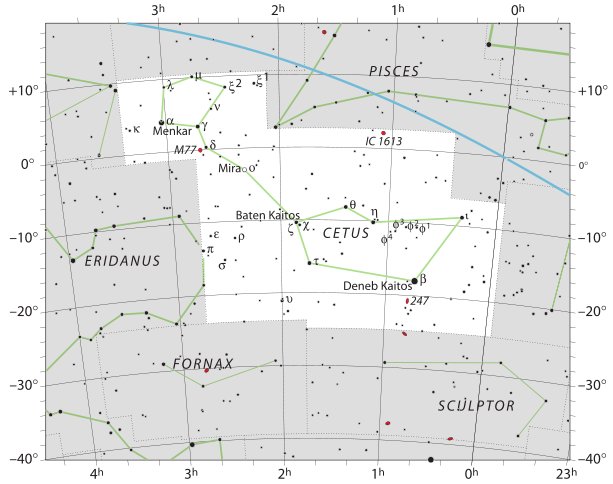
Take for example the star Menkar (α Ceti), which was culminating at midnight in December 21 according to Allen. I have connected Menkar by way of its right ascension with 'May 5 and the vero glyph in Gb8-12:

The distance to its midnight culmination in 'December 21 (Allen's dates could agree with the precessional time of the rongorongo texts) appears to be 355 - 125 = 230 days:

But if the heliacal rising of Menkar was in 'May 5, then we could expect Menkar to have been visible late at night just above the horizon in the east some days later, by arguing that Sun moves ahead among the constellations and that the heliacal risings will likeways go ahead to stars with higher and higher right ascension days. After a heliacal rising the star ought to be visible in the east late at night as if newborn.

I.e., we would have to wait - it might be concluded - a quarter of a year (corresponding to a quarter of the diurnal cycle of 24 hours) from 'May 5 in order to reach the date when Menkar would be seen positioned straight above at midnight on its way to the horizon in the west. 125 + 91 = 216, the Gregorian day number for August 4.

But when August 4 arrives, Earth must have changed its position relative to Sun, moved ahead in its orbit ca ¼ of its cycle. Therefore more days would be needed for Menkar to reach zenith at midnight.

However, counting the days ahead from 'December 21 to 'May 5 gives as a result (365 + 125) - 355 = 135. Therefore the distance between 'May 5 and 'December 21 should be 365 - 135 = 240 days, not 230 as suggested in the table above.

The riddle is seemingly solved by counting the day number for 'May 5 as 365 + 125 = 490, i.e. to regard 'May 5 as day 490 from 'January 1 in the previous year. 490 - 355 = 135 and 365 - 135 = 230:

But then also the day number for 'December 21 ought to be counted from 'January 1 in the previous year, because a midnight culmination comes later than a heliacal rising.

What comes first, the hen (culmination) or the egg (heliacal rising)? By counting with the 'hen' as the primary event and the 'egg' as the secondary event we can illustrate the situation as follows:

A hen is needed to produce an egg and when the chicken has emerged from its egg she will evolve into a hen. The zero symbol illustrates the egg.

Midnight culmination can thus be reached either by going forward, in the Menkar example with 135 nights, or by recalling in memory its position 230 nights earlier.