The step from Easter Island to Bolivia is not beyond what should be within the plausible reach of a great sea voyager of the past. Contacts could have gone equally well from South America to Easter Island as the other way around. And who would have wished to go only in one direction and never to come back?

If distance (in time and space) is an obstacle for credulity, then Pachamama is less of a problem than ancient Egypt. Let us therefore once again take a look at her:

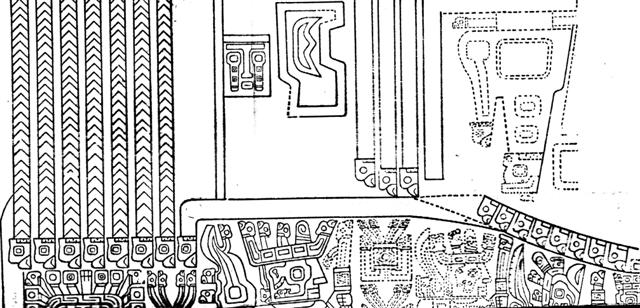
Her tresses are 14 in number, twice 7, but in this enlarged view of her right side it becomes evident that more should be considered. An extra 'tress' comes in horizontally from the right. And this one has no chevron marks but instead 8 bird heads on the front side, below the face of Pachamama. We should notice that the eyes of these 8 birds are drawn as single circles in contrast to the double-ring eyes of the nightside birds.

Instead of twice 7 tresses we apparently should think 2 * (7 + 1) = 16, because the patterns above are repeated mirror-wise on the left side of her head. The front side has twice 8 = 16 bird heads, the back side has twice 7 = 14 tresses, and on the border line between the front and the back side (below her ears) there is a pair of horizontal 'tresses' without chevron signs.

It could mean we ought to reconsider the meaning of 472 glyphs in the text of G. Maybe we should interpret them as 16 * 29.5 / 2 = 236 days on the front side (the bird heads), followed by 14 * 29.5 / 2 + 2 * 29.5 / 2 = 236 nights on the back side (including the border line area).

If fortnights were used as the basic Moon measure, then 'a greater fortnight' ought to have an even number of fortnights. Such a structure should be applied also if lunar synodic months were counted instead of months with 28 nights. A 'synodic fortnight' would be 29.5 / 28 * 14 = 14¾ nights long, and 14 such synodic fortnights will be equal to 14 * 14¾ = 206.5 nights. But presumably Moon should be counted twice, she has two faces, and 2 * 206.5 = 413 (= 14 * 29.5).